A Parameter-Free Action Collapse Threshold For Quantum Collapse

Author: David Werner

Abstract

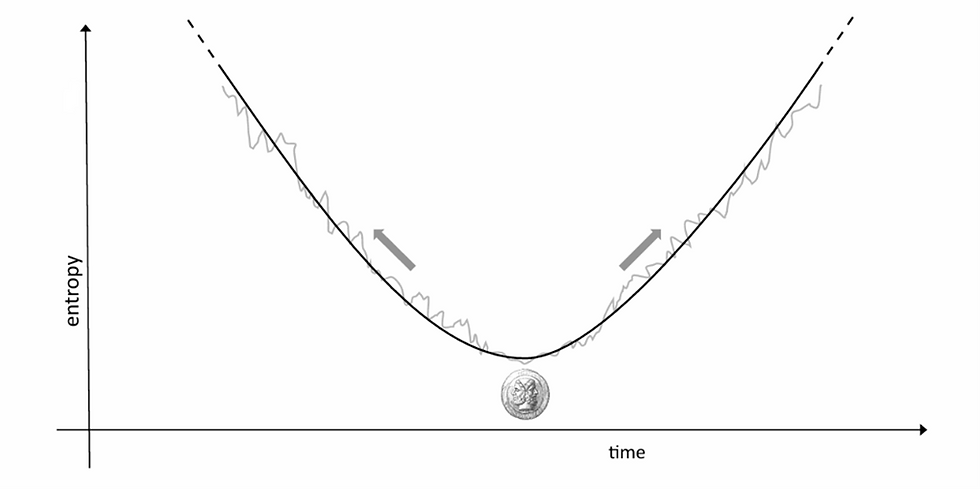
This paper proposes a speculative framework for a quantum collapse threshold based on irreversible action. Once the cumulative environmental action crosses a universal threshold, S=(e*h), quantum superpositions irreversibly transition into classical outcomes. This collapse threshold is motivated by thermodynamic principles: Euler’s number e describes the natural scale of irreversible entropy growth, and Planck’s constant h sets the fundamental quantum of action. This theory aims to prompt further discussion on the informational and thermodynamic underpinnings of quantum measurement. In this paper, a novel parameter-free and falsifiable law for quantum state reduction has been derived, which both predicts the Page curve and preserves the Born rule. This model provides a unified, testable framework connecting quantum measurement, thermodynamics, and information theory. 

Read online

Please click here to read this article online.

Download PDF

A Parameter-Free Action Collapse Threshold for Quantum Collapse - JYP.pdf

Download PDF • 1.62MB

References

[1] G.C. Ghirardi, A. Rimini, T. Weber, Unified Dynamics for Microscopic and Macroscopic Systems, Physical Review D 34, 470–491 (1986).

[2] R. Penrose, On Gravity's Role in Quantum State Reduction, General Relativity and Gravitation 28, 581–600 (1996).

[3] W.H. Zurek, Decoherence and the Transition from Quantum to Classical, Physics Today 44(10), 36–44 (1991).

[4] R. Landauer, Irreversibility and Heat Generation in the Computing Process, IBM Journal of Research and Development 5(3), 183–191 (1961).

[5] H.-P. Breuer and F. Petruccione, The Theory of Open Quantum Systems, Oxford University Press (2002).

[6] S. Weinberg, Collapse of the State Vector, Physical Review A 85, 062116 (2012).

[7] G. ’t Hooft, Dimensional Reduction in Quantum Gravity, arXiv:gr-qc/9310026 (1993).

[8] E. Verlinde, On the Origin of Gravity and the Laws of Newton, Journal of High Energy Physics 2011, 029 (2011).

[9] L. Diosi, Models for Universal Reduction of Macroscopic Quantum Fluctuations, Physical Review A 40, 1165–1174 (1989).

[10] J. von Neumann, Mathematical Foundations of Quantum Mechanics, Princeton University Press (1955).

[11] W.H. Zurek, Pointer Basis of Quantum Apparatus: Into What Mixture Does the Wave Packet Collapse?, Physical Review D 24, 1516–1525 (1981).

[12] M. Schlosshauer, Decoherence, the Measurement Problem, and Interpretations of Quantum Mechanics, Reviews of Modern Physics 76, 1267–1305 (2005).

[13] A. Bassi and G. C. Ghirardi, Dynamical Reduction Models, Physics Reports 379, 257–426 (2003).

[14] A. Bassi, K. Lochan, S. Satin, T. P. Singh and H. Ulbricht, Models of Wave-Function Collapse, Underlying Theories, and Experimental Tests, Reviews of Modern Physics 85, 471–527 (2013).

[15] M. Carlesso, A. Bassi, M. Paternostro and H. Ulbricht, Testing the Gravitational Field with Quantum Interferometry, New Journal of Physics 21, 093052 (2019).

[16] D. N. Page, Information in Black-Hole Radiation, Physical Review Letters 71, 3743–3746 (1993).

[17] A. Almheiri, T. Hartman, J. Maldacena, E. Shaghoulian and A. Tajdini, Entropy of Hawking Radiation as a Page Curve, Journal of High Energy Physics 2020 (09), 018 (2020).

[18] G. Lindblad, On the Generators of Quantum Dynamical Semigroups, Communications in Mathematical Physics 48, 119–130 (1976).